New Page 1

Combination To Form a Ring With Two Size of Brick

F o r m u l a

When Both Brick A and B are Radial and Outside Chords are equal (C =C) but Inside
Chord are Unique.

(1)

S = π D
C

(2)

Y = d - S E
e - E

When Brick B is a straight brick and Brick A is Redial, C = E

(3)

x = 2π T
C - E

(4)

y = πD - xC
c

When Brick A and B are Radial and Both Chord Dimension of A Differ From Those of B

(5)

Cx + cy = πD

(6)

Ex + ey = πd

When Calculating A Series of Combination The Following Formulas Are Convenient

(7)

X = J (g - D)
g - G

(8)

Y = J (g - D)
g - G

K e y T o S y m b o l

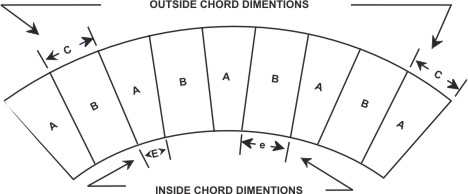
A	A radial brick used with a straight or another radial B, which turns a larger circle than A.
B	A straight brick or a radial brick which turns a larger circle than A, both a and B forming the same thickness of arch T.
T	The thickness of the arch formed by A and B.
C	The outside chord of brick A.
c	The outside chord of brick B.
E	The inside chord of brick A.
e	The inside chord of brick B.
D	Given outside diameter of desired ring.
d	Given inside diameter of desired ring.
G	Outside diameter of ring formed by radial brick A alone.
g	Outside diameter of ring formed by brick B alone if it is a radial.
J	Number of brick A to form a ring of outside diameter G.
j	Number of brick B to form a ring of outside diameter g.
x	Number of pieces of brick A, used with brick B to form a ring of outside diameter D
y	Number of pieces of brick B, used with brick A to form a ring of outside diameter D.
S	Total number of pieces of brick A and brick B to form a ring of outside diameter D.